이번 포스팅에서는 푸리에 사인 급수와 관련된 적분을 다뤄보겠습니다. Theorem. Let $f:(0,\infty)\to\mathbb{C}$ be a locally bounded Lebesgue-measurable function such that the improper integral $$ \int_{0}^{\infty} \frac{f(x)}{x} \, \mathrm{d}x = \lim_{\substack{a\to 0^+ \\ b\to\infty}} \int_{a}^{b} \frac{f(x)}{x} \, \mathrm{d}x $$ exists in $\mathbb{C}$. Then, for any $(a_n)_{n\geq 1}\subset\mathbb{C}$ satisfying $\sum_{n\geq1..
MSE를 뒤적이다가 슬쩍 본 계산인데, 예뻐서 가져와봅니다. We evaluate the integral $$ I = \int_{0}^{1} \int_{0}^{1} \frac{x}{1-(1-y^2) x^2} \, \mathrm{d}x\mathrm{d}y $$ in two ways. Integrating with respect to $x$ first, we get \begin{align*} I &= \int_{0}^{1} \frac{\log(1/y)}{1 - y^2} \, \mathrm{d}y = \sum_{n=0}^{\infty} \int_{0}^{1} y^{2n} \log(1/y) \, \mathrm{d}y = \sum_{n=0}^{\infty} \frac{1}{(2n+1)^2}. \end{align*..
In this posting, we compute the reproducing kernels of the Sobolev space $H^1([0,1])$ equipped with the inner product $$ \langle f, g \rangle = \int_{0}^{1} [ \overline{f(x)}g(x) + \overline{f'(x)}g'(x)] \,\mathrm{d}x. $$ In other words, we aim to find a function $K_a \in H^1([0, 1])$, for each $a \in [0, 1]$, such that $\langle K_a, f \rangle = f(a)$ for all $f \in H^1([0, 1])$. Assume there ex..
이번 포스팅에서는 뜬금없이 해석학 한 문제 다뤄보겠습니다. Theorem. Let $(X, d)$ be a compact metric space, and let $f:X\to X$ be a surjective $1$-Lipschitz function. Then $f$ is an isometry. Proof. Let $f^{\circ n}$ denote the $n$-fold composition of $f$, that is, $f^{\circ 0} = \mathrm{id}_{X}$ and $f^{\circ(n+1)} = f\circ f^{\circ n}$. By the assumption, the family $(f^{\circ n})_{n=1}^{\infty}$ is $1$-Lipschitz, henc..
Darboux method 란, 주어진 power series 혹은 Laurent series 의 계수들의 점근적인 행동을 조사할 때 유용한 방법 중 하나입니다. 이 방법은 간단하게 설명하자면 다음과 같습니다: 멱급수 $f(z) = \sum_{n=0}^{\infty} a_n z^n$ 가 $$ f(z) = g(z) + h(z), $$ 와 같은 꼴로 분해지며, 여기서 $g(z)$ 는 "조사하기 쉬운" 함수이며 $h(z)$ 는 "작은" 함수라고 합시다. 그러면 $f(z)$ 의 계수들 $(a_n)$ 의 점근적 행동이 $g(z)$ 의 계수들과 거의 비슷하다는 것이 이 방법의 요지입니다. 더욱 자세한 내용은 DLMF 의 해당 항목에서 확인하실 수 있습니다. 이 방법을 실제로 적용해보기 위하여, 다음과 같은 수열의 ..
제 Blogger 블로그에 몇 달 전에 올렸던 계산인데, 좀 마음에 들어서 여기서도 공유하고자 가져왔습니다. Calculation 1. We have $$ \int_{0}^{\infty} \frac{x^{8} - 4x^{6} + 9x^{4} - 5x^{2} + 1}{x^{12} - 10x^{10} + 37x^{8} - 42x^{6} + 26x^{4} - 8x^{2} + 1} \, \mathrm{d}x = \frac{\pi}{2}. $$ This is Problem 11148 of American Mathematical Monthly, Vol.112, April 2005. Proof. For each $f(z) \in \mathbb{C}[z]$, we define $f^*(z) = \overline{f(\..
드디어 qual 을 끝내고 나니, 이젠 개강이 내일이네요. 허허허, 쉴 틈이 없구나… 아래 명제는 제가 직접 푼 건 아니지만 그 내용이 마음에 들어서 한번 올려봅니다. Glasser 마스터 정리.[1] 상수 $\rho_{1}, \ldots, \rho_{n} > 0$ 와 $\alpha_{1}, \ldots, \alpha_{n}, \beta \in \mathbb{R}$ 이 주어졌다고 하자. 그러면 다음과 같이 정의된 함수 $$ \phi(x) = x - \beta - \sum_{i=1}^{n} \frac{\rho_{i}}{x - \alpha_{i}} $$ 는 $\mathbb{R}$ 위의 르벡 측도 $\operatorname{Leb}$ 를 보존한다. 즉, 임의의 르벡 측도가능한 집합 $E \subseteq \m..
정말 오랜만이네요. 요즘 정신도 없고 계산을 통 안하는 바람에... 명제. 다음 사실이 성립한다[1]: $$ \lim_{n\to\infty} \frac{(-1)^{n}n^{2}}{n!} \sum_{k=2}^{n} \binom{n}{k}(-1)^{k} k^{n-1} \log k = 2. $$ 증명은 여기에서 찾을 수 있습니다. Addendum. 뜬금없는 사족 하나. 일전에 일러스트들을 모아놓은 페이지들을 전에 시리즈로 올린 적이 있는데, 어떤 분이 저작권 문제를 지적하였기에 글을 내렸습니다. 저작권 문제 때문에 소란에 휘말리긴 싫네요... 널리 퍼뜨릴 수록 모두에게 이득이 되는 홍익정신의 결정체인 수학에나 더 매진해야겠습니다. 참고 문헌 r9m, Finding the limit of a sequence w..
간단한 문제 하나로 오랜만에 블로그의 정적이나 깨 볼까 합니다. 문제. $a \in \mathbb{R}$ 라고 하자. 또한 수열 $a_{n} \in \mathbb{C}$ 가 다음의 점화식을 만족시킨다고 하자. \begin{align*} a_{n} = a_{n-1} + \frac{a}{n} a_{n-2}, \quad n \geq 2. \end{align*} 그러면 아래와 같은 estimate 가 성립함을 보여라: \begin{align*} a_{n} = \mathcal{O}\left( n^{a} \right). \end{align*} 증명. Let $A_{n}$ and $B_{n}$ be sequences of $2\times 2$ matrices defined by \begin{align*} A_{n} ..
드디어 몇 년동안 짬짬히 고민하던 적분 문제에 상당한 진척을 보였습니다. 일단은 급한 일이 있어서, 결과 요약이랑 작성중인 증명 파일만 올립니다. 나중에 좀 더 살을 붙여야지요. Finally I made a significant progress in the integral problem I was struggling for some years. As I am busy now, I just present here a summarized result and the article containing the main proof. Theorem 1. For $ p, q, r > 0 $, define the generalized Ahmed's integral by \begin{equation} \label{int..
While calculating a specific problem, I succeeded in proving a more general problem. Proposition. For $0 < r < 1$ and $r < s$, the following holds:[1] \begin{equation} \label{eq_wts} \int_{-1}^{1} \frac{1}{x} \sqrt{\frac{1+x}{1-x}} \log \left| \frac{1 + 2rsx + (r^{2} + s^{2} - 1)x^{2}}{1 - 2rsx + (r^{2} + s^{2} - 1)x^{2}} \right| \, dx = 4\pi \arcsin r. \end{equation} Proof. We divide the proof ..
나흘 연휴의 절반이 지나가는 동안 한 거라곤 폐인짓밖에 없네…. 공부해야지~ Proposition. The following holds:[1] \begin{equation*} \tag{1} \int_{0}^{\frac{\pi}{2}} \arctan ( r \sin\theta ) \arctan ( s \sin\theta ) \, d\theta = \pi \chi_{2}(\alpha \beta), \end{equation*} where \begin{align*} \alpha = \frac{\sqrt{r^{2} + 1} - 1}{r}, \quad \beta = \frac{\sqrt{s^{2} + 1} - 1}{s} \end{align*} and $\chi_{2}$ is the Legendre chi funct..
Take Home Exam을 풀어야 하는데, 나는 이런 거나 계산하고 있을 뿐이고…. Proposition. The following holds:[1] \begin{align*} \sum_{n=1}^{\infty} \frac{H_{n}^{2}}{n^{2}} = \frac{17\pi^{4}}{360}. \end{align*} Proof. See the reference [1] below. Remark. This identity was first conjectured by Enrico Au-Yeung, a student of Jonathan Borwein, using computer search and the PSLQ algorithm, in 1993.[2] It is subsequently solved b..
밤새서 푼 적분 하나. 하라는 공부는 안 하고…! Proposition. The following holds:[1] \begin{align*} \int_{0}^{1} \frac{\log x \log(1-x) \log^{2}(1+x)}{x} \, dx = \frac{7}{8} \zeta(2)\zeta(3) - \frac{25}{16} \zeta(5). \end{align*} Proof. Below is my proof of the above identity. Step 1. Let \( I \) be the integral in question: \begin{align*} I &= \int_{0}^{1} \frac{\log x \log (1 - x) \log^{2} (1 + x)}{x} \, dx. \end..
과제하느라 밤새서 정신도 어지러운 와중에, 자라는 낮잠은 안 자고 40분동안 끙끙 싸매면서 푼 문제…. 진심으로 토할듯이 졸리니 이젠 정말 자러 가야겠네요. Proposition. The following holds:[1] \begin{align*} \int_{0}^{1} \log\left(1+\frac{\log^{2} x}{4\pi^{2}}\right)\frac{\log(1-x)}{x} \, dx = -\pi^{2} \left( 4\zeta'(-1) + \frac{2}{3} \right). \end{align*} Proof. See the reference [1] below. 풀이는 아래 참고문헌 [1]에 있습니다. 요즘 블로그로 풀이를 옮기기가 너무 귀찮아서 그냥 링크로... 허허 References..
수업을 듣다가, 꽤나 흥미로운 사실인 것 같아서 그냥 정리해둡니다. Proposition. Define elementary symmetric polynomials of $n$ variables $\Lambda = \{ \lambda_{1}, \cdots, \lambda_{n} \}$ by \begin{align*} c_{0} = 1, \quad c_{k} = \sum_{\substack{X \subset \Lambda \\ |X| = k}} \prod_{\lambda \in X} \lambda \quad (1 \leq k \leq n) \end{align*} and similarly we define \begin{align*} s_{k} = \sum_{j=1}^{n} \lambda_{j}^{k} \qua..
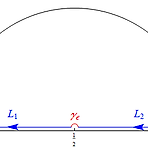 오늘의 계산 57 - Calculation Suite
오늘의 계산 57 - Calculation Suite
그동안 여기저기 싸지른 잡다한 계산들을 한 번 정리해볼까 합니다. Problem #. Show that \begin{equation*} \int_{0}^{\frac{\pi}{2}} \log \left(x^{2} + \log^{2}(\cos x) \right) \, \mathrm{d}x = \pi \log \log 2. \tag{1} \end{equation*} This problem is from [IS1]. Solution. Let $I$ denote the integral in $\text{(1)}$. By recalling the identity \begin{align*} x^{2} + \log^{2} (\cos x) = \left| \log \left( \frac{1+e^{2ix}}{2} \rig..
Here I am going to introduce some easy results on some criteria for interchanging the order of integration which are not covered by the classical Fubini theorem. Though both statements and proofs are weak and easy, it often reduces our burden to large extent. Let $f$ be a locally integrable function on $(0, \infty)$. That is, $f$ is a measurable function which is integrable on any compact subset..
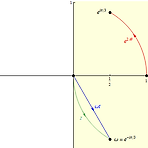 오늘의 계산 56 - An infinite summation involving binomial coefficient
오늘의 계산 56 - An infinite summation involving binomial coefficient
Problem 1. Prove that[각주:1] \begin{equation*} \sum_{n=1}^{\infty} \frac{1}{n^4 \binom{2n}{n}} = \frac{17\pi^4}{3240}. \tag{1} \end{equation*} Proof. We divide the proof into several steps. 1. Reduction to an integral representation Let $S$ denote the summation in question. By using the Lemma 1 in Today's Calculation 29, we can represent $S$ as an integral. Then by the successive application of i..
Problem 1. Show that[각주:1] \begin{equation*} \int_{0}^{\infty} x \left\{ (2S(x) - 1)^2 + (2C(x) - 1)^2 \right\}^{2} \, \mathrm{d}x = \frac{16 \log 2 - 8}{\pi^2}, \tag{1} \end{equation*} where $S(x)$ and $C(x)$ denote the Fresnel integrals defined by \begin{align*} S(x) = \int_{0}^{x} \sin \left( \tfrac{1}{2} \pi t^2 \right) \, \mathrm{d}t \quad \text{and} \quad C(x) = \int_{0}^{x} \cos \left( \t..
- Total
- Today
- Yesterday
- infinite summation
- 계산
- 보컬로이드
- binomial coefficient
- 노트
- 대수기하
- Fourier Transform
- 이항계수
- 루카
- Beta function
- 제타함수
- 편미방
- 미쿠
- 해석학
- 적분
- Coxeter
- 오일러 적분
- Zeta function
- 푸리에 변환
- 렌
- Euler integral
- 감마함수
- 린
- 무한급수
- 수학
- Integral
- Euler constant
- 유머
- Gamma Function
- 오일러 상수
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| 8 | 9 | 10 | 11 | 12 | 13 | 14 |
| 15 | 16 | 17 | 18 | 19 | 20 | 21 |
| 22 | 23 | 24 | 25 | 26 | 27 | 28 |
| 29 | 30 |
