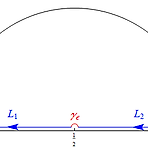
MSE를 뒤적이다가 슬쩍 본 계산인데, 예뻐서 가져와봅니다. We evaluate the integral $$ I = \int_{0}^{1} \int_{0}^{1} \frac{x}{1-(1-y^2) x^2} \, \mathrm{d}x\mathrm{d}y $$ in two ways. Integrating with respect to $x$ first, we get \begin{align*} I &= \int_{0}^{1} \frac{\log(1/y)}{1 - y^2} \, \mathrm{d}y = \sum_{n=0}^{\infty} \int_{0}^{1} y^{2n} \log(1/y) \, \mathrm{d}y = \sum_{n=0}^{\infty} \frac{1}{(2n+1)^2}. \end{align*..
제 Blogger 블로그에 몇 달 전에 올렸던 계산인데, 좀 마음에 들어서 여기서도 공유하고자 가져왔습니다. Calculation 1. We have $$ \int_{0}^{\infty} \frac{x^{8} - 4x^{6} + 9x^{4} - 5x^{2} + 1}{x^{12} - 10x^{10} + 37x^{8} - 42x^{6} + 26x^{4} - 8x^{2} + 1} \, \mathrm{d}x = \frac{\pi}{2}. $$ This is Problem 11148 of American Mathematical Monthly, Vol.112, April 2005. Proof. For each $f(z) \in \mathbb{C}[z]$, we define $f^*(z) = \overline{f(\..
드디어 몇 년동안 짬짬히 고민하던 적분 문제에 상당한 진척을 보였습니다. 일단은 급한 일이 있어서, 결과 요약이랑 작성중인 증명 파일만 올립니다. 나중에 좀 더 살을 붙여야지요. Finally I made a significant progress in the integral problem I was struggling for some years. As I am busy now, I just present here a summarized result and the article containing the main proof. Theorem 1. For $ p, q, r > 0 $, define the generalized Ahmed's integral by \begin{equation} \label{int..
While calculating a specific problem, I succeeded in proving a more general problem. Proposition. For $0 < r < 1$ and $r < s$, the following holds:[1] \begin{equation} \label{eq_wts} \int_{-1}^{1} \frac{1}{x} \sqrt{\frac{1+x}{1-x}} \log \left| \frac{1 + 2rsx + (r^{2} + s^{2} - 1)x^{2}}{1 - 2rsx + (r^{2} + s^{2} - 1)x^{2}} \right| \, dx = 4\pi \arcsin r. \end{equation} Proof. We divide the proof ..
나흘 연휴의 절반이 지나가는 동안 한 거라곤 폐인짓밖에 없네…. 공부해야지~ Proposition. The following holds:[1] \begin{equation*} \tag{1} \int_{0}^{\frac{\pi}{2}} \arctan ( r \sin\theta ) \arctan ( s \sin\theta ) \, d\theta = \pi \chi_{2}(\alpha \beta), \end{equation*} where \begin{align*} \alpha = \frac{\sqrt{r^{2} + 1} - 1}{r}, \quad \beta = \frac{\sqrt{s^{2} + 1} - 1}{s} \end{align*} and $\chi_{2}$ is the Legendre chi funct..
Take Home Exam을 풀어야 하는데, 나는 이런 거나 계산하고 있을 뿐이고…. Proposition. The following holds:[1] \begin{align*} \sum_{n=1}^{\infty} \frac{H_{n}^{2}}{n^{2}} = \frac{17\pi^{4}}{360}. \end{align*} Proof. See the reference [1] below. Remark. This identity was first conjectured by Enrico Au-Yeung, a student of Jonathan Borwein, using computer search and the PSLQ algorithm, in 1993.[2] It is subsequently solved b..
밤새서 푼 적분 하나. 하라는 공부는 안 하고…! Proposition. The following holds:[1] \begin{align*} \int_{0}^{1} \frac{\log x \log(1-x) \log^{2}(1+x)}{x} \, dx = \frac{7}{8} \zeta(2)\zeta(3) - \frac{25}{16} \zeta(5). \end{align*} Proof. Below is my proof of the above identity. Step 1. Let \( I \) be the integral in question: \begin{align*} I &= \int_{0}^{1} \frac{\log x \log (1 - x) \log^{2} (1 + x)}{x} \, dx. \end..
과제하느라 밤새서 정신도 어지러운 와중에, 자라는 낮잠은 안 자고 40분동안 끙끙 싸매면서 푼 문제…. 진심으로 토할듯이 졸리니 이젠 정말 자러 가야겠네요. Proposition. The following holds:[1] \begin{align*} \int_{0}^{1} \log\left(1+\frac{\log^{2} x}{4\pi^{2}}\right)\frac{\log(1-x)}{x} \, dx = -\pi^{2} \left( 4\zeta'(-1) + \frac{2}{3} \right). \end{align*} Proof. See the reference [1] below. 풀이는 아래 참고문헌 [1]에 있습니다. 요즘 블로그로 풀이를 옮기기가 너무 귀찮아서 그냥 링크로... 허허 References..
 오늘의 계산 57 - Calculation Suite
오늘의 계산 57 - Calculation Suite
그동안 여기저기 싸지른 잡다한 계산들을 한 번 정리해볼까 합니다. Problem #. Show that \begin{equation*} \int_{0}^{\frac{\pi}{2}} \log \left(x^{2} + \log^{2}(\cos x) \right) \, \mathrm{d}x = \pi \log \log 2. \tag{1} \end{equation*} This problem is from [IS1]. Solution. Let $I$ denote the integral in $\text{(1)}$. By recalling the identity \begin{align*} x^{2} + \log^{2} (\cos x) = \left| \log \left( \frac{1+e^{2ix}}{2} \rig..
 오늘의 계산 56 - An infinite summation involving binomial coefficient
오늘의 계산 56 - An infinite summation involving binomial coefficient
Problem 1. Prove that[각주:1] \begin{equation*} \sum_{n=1}^{\infty} \frac{1}{n^4 \binom{2n}{n}} = \frac{17\pi^4}{3240}. \tag{1} \end{equation*} Proof. We divide the proof into several steps. 1. Reduction to an integral representation Let $S$ denote the summation in question. By using the Lemma 1 in Today's Calculation 29, we can represent $S$ as an integral. Then by the successive application of i..
Problem 1. Show that[각주:1] \begin{equation*} \int_{0}^{\infty} x \left\{ (2S(x) - 1)^2 + (2C(x) - 1)^2 \right\}^{2} \, \mathrm{d}x = \frac{16 \log 2 - 8}{\pi^2}, \tag{1} \end{equation*} where $S(x)$ and $C(x)$ denote the Fresnel integrals defined by \begin{align*} S(x) = \int_{0}^{x} \sin \left( \tfrac{1}{2} \pi t^2 \right) \, \mathrm{d}t \quad \text{and} \quad C(x) = \int_{0}^{x} \cos \left( \t..
Problem 1. Show that[각주:1] the product \begin{equation*} P = \left( \frac{2}{1} \right)^{\frac{1}{8}} \left( \frac{2 \cdot 2}{1 \cdot 3} \right)^{\frac{3}{16}} \left( \frac{2 \cdot 2 \cdot 2 \cdot 4}{1 \cdot 3 \cdot 3 \cdot 3} \right)^{\frac{6}{32}} \left( \frac{2 \cdot 2 \cdot 2 \cdot 2 \cdot 4 \cdot 4 \cdot 4 \cdot 4}{1 \cdot 3 \cdot 3 \cdot 3 \cdot 3 \cdot 3 \cdot 3 \cdot 5} \right)^{\frac{10..
Here we want to calculate an integral related to our earlier calculation. Problem. Prove that \begin{equation} \label{eqn:wts} \int_{0}^{1} \left( \frac{1}{\log x} + \frac{1}{1-x} \right)^2 \, dx = \log (2\pi) - \frac{3}{2}. \end{equation} 1st Proof. Note that $$ \frac{1}{1-x} + \frac{1}{\log x} = \int_{0}^{1} \frac{1 - x^{t}}{1-x} \, dt. $$ Then by Tonelli's theorem, we have \begin{align*} \int..
이번 계산에서는, 마지막 부분에 제가 깔끔하게 처리하지 못한 부분을 다른 분의 도움을 얻어 깔끔하게 처리해보았습니다. 어떤 분은 복소로 풀어내기도 했는데, 이 풀이 역시 상당히 마음에 와닿더군요. Problem. Let $(u_n)$ be the sequence defined by \begin{equation} \label{eqn:recur} \frac{u_1}{n} + \frac{u_2}{n-1} + \cdots + \frac{u_{n-1}}{2} + u_n = 1. \end{equation} Find the asymptotic behavior of $u_n$. Solution. We introduce the generating function $U(x)$ of $(u_n)$ given by $$U(x..
이전에 비슷한 적분을 실해석적으로 푼 적이 있는데, 이번에는 깔끔하게 복소로 계산해보았습니다. 제 복소적분 실력도 못 써먹을 수준은 아니군요. Problem. Prove that[1] $$ \int_0^{\pi/2} \frac{\log(\cos x)}{x^2+\log^2(\cos x)} \, dx = \frac{\pi}{2}\left(1-\frac{1}{\log 2}\right) $$ Proof. Note that for $|x| < \frac{\pi}{2}$, we have $$ \frac{\log\cos x}{\log^2 \cos x + x^2} = \Re \frac{1}{\log\left( \frac{1+e^{2ix}}{2} \right)}. $$ Thus if $I$ denotes the give..
Here are chosen ones from my 50+ε calculations from the Today's Calculation archive, which are polished and supplemented. Any feedback is greatly welcomed. Table of Contents Preliminary Some materials on analysis Mathematical Constants Riemann Zeta function Gamma function and Polygamma functions Beta function Polylogarithms and related functions Today’s Calculation Derivation of $\zeta(2n)$ An i..
Enjoy this calculation and have a good day! Problem. For $\alpha > 0$, examine the following limit \begin{equation}\label{eqn:wts} \lim_{n\to\infty} e^{-\alpha\sqrt{n}} \sum_{k=0}^{n-1} 2^{-n-k} \binom{n-1+k}{k} \sum_{m=0}^{n-1-k}\frac{(\alpha\sqrt{n})^m}{m!}. \end{equation} Solution. Let $A_n$ denote the formula inside the limit \eqref{eqn:wts}. By noting that the double summation is taken for ..
Today I want to deal with a problem which does not lie in analysis. It would be easy for those who are adapted to algebra, but for those with algebra trauma like me, it was rather a challenge. Problem. Let $m$ be an integer and $(u_n)$ be a sequence defined by \begin{equation}\label{eqn:recur} u_0 = 1, \quad u_1 = m \quad \text{and} \quad u_{n+2} = mu_{n+1} + u_n. \end{equation} Prove that for a..
Problem 1. Evaluate the following integral.[1] \begin{equation}\label{prob:wts} \int_{0}^{\infty} \frac{u \log(a^2+u^2)}{e^{2\pi u} - 1} \; du \end{equation} Proof. Let $I(a)$ denote the given integral. Then we have \begin{align*}I(0) & = 2 \int_{0}^{\infty} \frac{u \log u}{e^{2\pi u} - 1} \; du \\ & = \frac{1}{2\pi^2} \int_{0}^{\infty} \frac{x (\log x - \log (2\pi))}{e^{x} - 1} \; dx \qquad (x ..
Problem. Prove that[1] \begin{equation}\label{prob:wts} \int_{0}^{\frac{\pi}{2}} \frac{x^2}{x^2 + \log^2(2\cos x)} \; dx = \frac{\pi}{8}\left( 1 - \gamma + \log(2\pi) \right). \end{equation} Proof. Here I refer to the following identity \begin{equation}\label{eq:01} \binom{\alpha}{\omega} = \frac{1}{2\pi} \int_{-\pi}^{\pi} \left(1 + e^{i\theta}\right)^{\alpha} e^{-i\omega \theta} \; d\theta, \en..
- Total
- Today
- Yesterday
- 미쿠
- 렌
- 수학
- 보컬로이드
- 루카
- 적분
- 감마함수
- 푸리에 변환
- Coxeter
- Euler constant
- Beta function
- Euler integral
- infinite summation
- Integral
- 린
- 무한급수
- Gamma Function
- Zeta function
- 제타함수
- 해석학
- 오일러 적분
- binomial coefficient
- 이항계수
- 편미방
- 오일러 상수
- 계산
- 대수기하
- Fourier Transform
- 노트
- 유머
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | ||
| 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| 13 | 14 | 15 | 16 | 17 | 18 | 19 |
| 20 | 21 | 22 | 23 | 24 | 25 | 26 |
| 27 | 28 | 29 | 30 | 31 |
