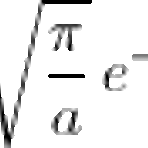Problem. Show that \begin{equation}\label{prob:wts} \int_{0}^{\infty} \frac{\cos ax}{x} \, \frac{\sinh\beta x}{\cosh\gamma x} \, dx = \frac{1}{2} \, \log \left( \frac{\cosh\frac{a\pi}{2\gamma}+\sin\frac{\beta\pi}{2\gamma}}{\cosh\frac{a\pi}{2\gamma}-\sin\frac{\beta\pi}{2\gamma}} \right) \end{equation} for $|\Re \beta| < \Re \gamma$. (Original Problem by Doe John, in his posting [1].) To prove \eq..
 Too Easy To Calculate
Too Easy To Calculate
The purpose of this posting is to encourage this blog not to suffer from stagnation, though I'm not sure whether it will work or not. Here, we introduce a very easy calculation, though in a slightly unfamiliar fashion. \begin{align*} \int\sqrt{\tan x}\;dx &=\int\frac{2t^2}{t^4+1}\;dt\qquad(t=\sqrt{\tan x})\\ &=\int\frac{2}{t^2+t^{-2}}\;dt\\ &=\int\left(\frac{1-t^{-2}}{\left(t+t^{-1}\right)^2-2}+..
 Numerical Solution of 1-D Heat Equation
Numerical Solution of 1-D Heat Equation
확률론적인 방법으로 1-D heat equation을 푸는 방법을 간단하게 Mathematica로 구현해보았습니다. 아래의 그래프 는 $t = 0$ 일 때 $[-1, 1]$에서 $1$의 값을 갖고 나머지 지점에서는 모두 $0$인 초기조건이 주어졌을 때, \begin{equation}\label{heat_eq} \frac{\partial u}{\partial t} = \frac{1}{2}\Delta u \end{equation} 를 Monte-Carlo method로 계산하여 $(t, x) \in [0, 1] \times [-2, 2]$ 영역에서 밀도그래프로 그린 것입니다. (왜 굳이 \eqref{heat_eq}에서 $\Delta$ 앞에 $\frac{1}{2}$를 붙였냐면, Brownian motion의 ..
오늘의 계산 시리즈로 여러분들을 다시 찾아뵙는 것은 정말 오랜만이네요. 거의 반 년 가까이 계산을 놓고 있었는데, 오늘 좋은 떡밥을 하나 줏어서 열심히 머리굴린 덕에 계산해낼 수 있었습니다. 한 3시간 정도 매달린 것 같네요. 아, 어지러워... 진리의 미쿠로 다시 회복하고 확률미방이나 공부해야겠네요. Problem. Find the value of the integral \begin{equation*} \int_{0}^{1} \frac{\log \log (1/x)}{1+x^2} \; dx. \end{equation*} Solution. By the substitution \( x = e^{-t} \), we find that \begin{align*} \int_{0}^{1} \frac{(\log (1/..
이런 거라도 올려야 블로그가 정체되지 않겠지요? 으헣헣 ;ㅅ; Tonelli's theorem enables us to exchange the order of integration and summation of a sequence of nonnegative functions. Thus for \( 0 < r < 1 \), \begin{align*} \sum_{n=0}^{\infty} \frac{r^n}{n!} \int_{0}^{\infty} x^n e^{-x} \; dx & = \int_{0}^{\infty} \sum_{n=0}^{\infty} \frac{(rx)^n}{n!} e^{-x} \; dx = \int_{0}^{\infty} e^{-(1 - r)x} \; dx \\ & = \frac{1}{1 -..
고딩때 독서실에서 하라는 시험공부는 안 하고 노트에 끄적였던 내용이 문득 떠올라서, 조금 다듬어서 올려봅니다. Definition 1.네 행렬 \(U\), \(I\), \(J\), \(K\)를 다음과 같이 정의한다. \begin{equation} U = \begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix}, \quad I = \begin{pmatrix} 0 & -1 \\ 1 & 0 \end{pmatrix}, \quad J = \begin{pmatrix} 1 & 0 \\ 0 & -1 \end{pmatrix}, \quad K = \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix}. \end{equation} 그러면 다음과 같은 관찰을 할 수 있습니다. ..
(2011 SEEMOUS Mathematical Competition, Problem 3) Show that for any three unit vectors \( \hat{a}\), \(\hat{b}\) and \(\hat{c}\), \begin{equation*} \langle \hat{a}, \hat{c} \rangle^2 + \langle \hat{b}, \hat{c} \rangle^2 \leq 1 + | \langle \hat{a}, \hat{b} \rangle| \end{equation*} Proof. Decompose \begin{align*} \hat{a} &= \cos \alpha \, \hat{c} + \sin \alpha \, \hat{e}_{1}, \hat{b} &= \cos \bet..
명색이 수학과 잡담을 위한 블로그인데 요즘 너무 잡담만 했으니, 땜빵용 허접 문제 몇개 올려봅니다. 첫 번째 건 몇년 전에 풀어서 이전 블로그에도 올린 내용이니까, 사실상 한 문제밖에 포스팅하지 않은 셈이네요. 으흐흐흐... Problem 1. Let \( k \) be a field of characteristic \( \neq 2 \), and \( A \) and \( B \) be two 2 by 2 matrices on \( k \). Then prove that \(A^{2} - 2AB + B^{2} = O\) implies \((A-B)^{2} = O\). Solution. Let \( C = A - B \). Then we have to show that \( C^{2} = O \). By..
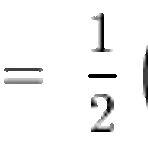 조만간 포스팅할 계산들 (Calculations To Be Posted)
조만간 포스팅할 계산들 (Calculations To Be Posted)
Calculation 1. For a positive number \( a \), we have Calculation 2. Calculation 3. 관심있는 분들도 한 번 같이 풀어보시라고 올려봤습니다. 두 번째 계산은 풀이가 이미 적혀있지만, 뭐 어차피 일반화된 결과를 올릴 계획이라 상관 없습니다. p.s. 제가 폐인인 이유를 깨달았습니다. 전 컴퓨터 중독이었던 것입니다! 으아아악, 삼성장학금 빨리 신청해야 되는데 ㅇ
특정한 조건을 만족시키는 함수의 iteration에 대한 간단한 estimation입니다. Assumptions. Suppose for \( a \neq 0 \), there is \( m > 0 \) such that \[ f(x) = x \left( 1 - (a + o(1)) x^{m} \right) \] near \( x = 0 \). Also, suppose the sequence \( (x_k) \) given by the recurrence relation \[ x_{k+1} = f(x_k) \] is positive and vanishes as \( k \to \infty \). Calculation 1. By simple calculation, we have \[ \frac{1}{x_{k+..
 오늘의 계산 43 - Calculations of Four Integrals
오늘의 계산 43 - Calculations of Four Integrals
Here I post my solutions to the announced four integrals. I tacitly assumed some formulas to be granted, so to reduce unnecessary calculations that are attainable in regular courses in analysis. One exception to this convention is the detailed proofs for the identity \begin{equation*} \int_{0}^{\infty} \frac{\cos x}{x^2 + \beta^2} \; dx = \frac{\pi}{2\beta} e^{-\beta} \end{equation*} for $\beta ..
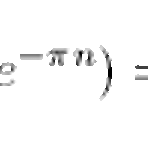 오늘의 계산 42 - A Product related to Dedekind eta function
오늘의 계산 42 - A Product related to Dedekind eta function
Problem. Show the identity \begin{equation} \label{pb:wts} \prod_{n=1}^{\infty} \left(1 + e^{- \pi n}\right) = 2^{-\frac{1}{8}} e^{\frac{\pi}{24}}. \end{equation} Proof. This is related to the famous Dedekind eta function $\eta(s)$, which is defined by \begin{equation*} \eta(\tau) = e^{\frac{\pi i \tau}{12}} \prod_{n=1}^{\infty} \left( 1 - e^{2\pi i n \tau} \right), \end{equation*} although we d..
 오늘의 계산 41 - Realizing Nonsensical (in progress)
오늘의 계산 41 - Realizing Nonsensical (in progress)
오늘은, 소설로 치면 그냥 커뮤니티에 의미도 없이 순간 흥해서 싸질러보는 무의미한 줄거리에 가까운 계산입니다. 뭔가 '아, 이런 적분에 의미를 줄 수 있을까' 하는 생각에서 출발해봤는데, 그냥 뭐 그렇네요 -_-;; Let $ f $ be a measurable function on $ (0, \infty) $. If \begin{equation} \label{def:ctr} \int_{h}^{\infty} f(x) \, \frac{dx}{x} = -\log h + c + o(1) \quad \text{as} \ h \to 0^{+} \end{equation} for some complex number $ c $, then we call $ c $ the center of $ f $ and denote ..
Today we are going to prove the following equality. Problem. Show that Proof. Let denote this integral hereafter. To achieve this, we first observe by double-angle formula that Here, we used the transformation for each step. Then by symmetry, this is written as Then by the identity we have Then we make change of variable to obtain where denote the counterclockwised unit circle centered at the or..
Lemma 1. (Complex Gaussian Integral) Suppose $s \in \mathbb{C}$ satisfies $s \neq 0$ and $\Re (s) \geq 0$. Then \[ \int_{-\infty}^{\infty} e^{-s x^2} \, dx = \sqrt{\frac{\pi}{s}}, \] where $\sqrt{z} = \exp(\frac{1}{2} \text{Log} z)$ denotes the principal square root of $z \in \mathbb{C} - (-\infty, 0)$. Pf. For $\Re (s) > 0$, the change of variable with $x = \sqrt{y}$ yields \[ \int_{-\infty}^{\..
Here we are going to present some conditions that enables us to interchange the order of integration which is often not covered by classic Fubini's Theorem or its variants. We assume readers are familiar with basic theory of Lebesgue-Stieltjes integration. (Refer to this article for the definition of terms that are used throughout this post) 1. Preliminaries We start with two definitions that sl..
 오늘의 계산 39 - Abel's Integral
오늘의 계산 39 - Abel's Integral
Today's integral is so called Abel's integral, which is given by Problem. \begin{equation} \label{eq:prob} \int_{0}^{\infty} \frac{t \; dt}{(e^{\pi t} - e^{-\pi t})(t^2 + 1)} \end{equation} Now our aim is to evaluate \eqref{eq:prob} in closed form. We preliminarily introduce a function that will play a key role throughout the calculation. Let $k$ be a nonnegative integer. Then we define polygamm..
- Total
- Today
- Yesterday
- 편미방
- 노트
- 오일러 적분
- Fourier Transform
- Zeta function
- 미쿠
- 린
- 해석학
- 보컬로이드
- 푸리에 변환
- Beta function
- 감마함수
- infinite summation
- 렌
- 무한급수
- 제타함수
- Coxeter
- 이항계수
- 계산
- 유머
- Integral
- binomial coefficient
- Gamma Function
- 대수기하
- Euler constant
- 적분
- 루카
- 수학
- Euler integral
- 오일러 상수
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| 8 | 9 | 10 | 11 | 12 | 13 | 14 |
| 15 | 16 | 17 | 18 | 19 | 20 | 21 |
| 22 | 23 | 24 | 25 | 26 | 27 | 28 |
| 29 | 30 |