 스프라이트(Sprite), 베일에 가려진 구름 위의 번개
스프라이트(Sprite), 베일에 가려진 구름 위의 번개
여러분들은 혹시 스프라이트(sprite)라고 들어보셨는지요? 사이다나 요정을 이야기하는 것이 아닙니다. 스프라이트는 폭풍우 번개의 위에서 일어나는 TLE(Transient Luminous Event; 고층대기 극한방전현상) 중에서 가장 대표적인 현상으로, 쉽게 말하면 매우 짧은 시간 안에 구름 위에서 일어나는 번개입니다. 사실 우리는 번개가 구름에서 땅으로 내려온다고 알고 있습니다. 좀 더 자세히 아는 사람들은, 번개가 평균 0.5초 정도 지속되며, 음전하를 띠고, 평균 3만 암페어의 전류를 띤다는 것을 알고 있을 겁니다. 하지만 땅과 구름 사이에서 일어나는 번개만 해도 여러 종류가 있습니다. 땅에서 구름으로 치는 번개도 있으며, 이 번개는 후에 다른 번개들을 유도하기 때문에 상당히 위험합니다. 또한 전..
 오늘의 계산 41 - Realizing Nonsensical (in progress)
오늘의 계산 41 - Realizing Nonsensical (in progress)
오늘은, 소설로 치면 그냥 커뮤니티에 의미도 없이 순간 흥해서 싸질러보는 무의미한 줄거리에 가까운 계산입니다. 뭔가 '아, 이런 적분에 의미를 줄 수 있을까' 하는 생각에서 출발해봤는데, 그냥 뭐 그렇네요 -_-;; Let $ f $ be a measurable function on $ (0, \infty) $. If \begin{equation} \label{def:ctr} \int_{h}^{\infty} f(x) \, \frac{dx}{x} = -\log h + c + o(1) \quad \text{as} \ h \to 0^{+} \end{equation} for some complex number $ c $, then we call $ c $ the center of $ f $ and denote ..
Today we are going to prove the following equality. Problem. Show that Proof. Let denote this integral hereafter. To achieve this, we first observe by double-angle formula that Here, we used the transformation for each step. Then by symmetry, this is written as Then by the identity we have Then we make change of variable to obtain where denote the counterclockwised unit circle centered at the or..
Lemma 1. (Complex Gaussian Integral) Suppose $s \in \mathbb{C}$ satisfies $s \neq 0$ and $\Re (s) \geq 0$. Then \[ \int_{-\infty}^{\infty} e^{-s x^2} \, dx = \sqrt{\frac{\pi}{s}}, \] where $\sqrt{z} = \exp(\frac{1}{2} \text{Log} z)$ denotes the principal square root of $z \in \mathbb{C} - (-\infty, 0)$. Pf. For $\Re (s) > 0$, the change of variable with $x = \sqrt{y}$ yields \[ \int_{-\infty}^{\..
Here we are going to present some conditions that enables us to interchange the order of integration which is often not covered by classic Fubini's Theorem or its variants. We assume readers are familiar with basic theory of Lebesgue-Stieltjes integration. (Refer to this article for the definition of terms that are used throughout this post) 1. Preliminaries We start with two definitions that sl..
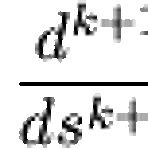 오늘의 계산 39 - Abel's Integral
오늘의 계산 39 - Abel's Integral
Today's integral is so called Abel's integral, which is given by Problem. \begin{equation} \label{eq:prob} \int_{0}^{\infty} \frac{t \; dt}{(e^{\pi t} - e^{-\pi t})(t^2 + 1)} \end{equation} Now our aim is to evaluate \eqref{eq:prob} in closed form. We preliminarily introduce a function that will play a key role throughout the calculation. Let $k$ be a nonnegative integer. Then we define polygamm..
 오늘의 계산 38 - Coxeter's Integrals (2)
오늘의 계산 38 - Coxeter's Integrals (2)
오늘 올리는 계산은 Coxeter's Integrals라는 적분의 계산입니다. 사실 이 적분을 처음 접한 것은 몇 년 전이지만, 그 동안은 번번히 계산에 실패했었습니다. 그러던 중, 지난 번 「오늘의 계산 37」의 접근법을 기본으로 하고, 아크코사인을 적절히 변형하는 방법을 발견해내어 이렇게 계산에 성공하게 되었습니다. 으, 참으로 긴 여정이었습니다만 그만큼 뿌듯하긴 하네요. Problem (Coxeter's integrals). Verify the following identities. \begin{align} \int_{0}^{\frac{\pi}{2}} \cos^{-1} \left( \frac{\cos x}{1+2\cos x}\right) \, dx & = \frac{5}{4} \zeta(2) \la..
 오늘의 계산 37 - Coxeter's Integrals (1)
오늘의 계산 37 - Coxeter's Integrals (1)
Today's integral is a famous one. $$ \int_{0}^{\frac{\pi}{4}} \arctan\sqrt{\frac{\cos2\theta}{2\cos^2\theta}} \, \mathrm{d}\theta = \frac{\pi^2}{24}. $$ To calculate this, we first note that \begin{gather*} \arctan(\alpha) = \int_{0}^{1} \frac{\alpha}{1+\alpha^2 x^2} \, \mathrm{d}x, \tag{1} \\ \int_{0}^{\infty} \frac{\mathrm{d}x}{(x^2+\alpha^2)(x^2 + \beta^2)}=\frac{\pi}{2\alpha\beta(\alpha+\bet..
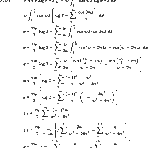 오늘의 계산 36 - Integration of a Fejer kernel-like thing
오늘의 계산 36 - Integration of a Fejer kernel-like thing
…을 가장한, 옛날에 AoPS에 답변으로 올렸지만 티스토리로 퍼오기 매우 귀찮아서 그냥 방치했던 계산 하나를 올려봅니다. 다른 꼴의 삼각함수 적분에도 쓸 수 있는 테크닉이 아닐까 해서 이렇게 올려봅니다. Today's integral we are going to evaluate is a famous one, . Solution 1 (by elementary calculus). It is clear that . To determine for , we consider the difference for . Some trigonometric identities show that Since it follows that and for . Therefore for all . Solution 2 (by complex ..
오늘 계산은 지금까지 MathLinks에 답변했던 계산들 중 2개를 추려서 올려본 것입니다. 이번에 계산할 적분은 아래 적분입니다. 특별한 설명 없이 쭉 이어나가겠습니다. \begin{align*} I & = \int_{0}^{1}\frac{\sqrt{x-x^{2}}}{(1+x^{2})^{2}}\, \mathrm{d}x \\ &= \int_{0}^{\infty}\frac{\sqrt{y}(1+y)}{(1+(1+y)^{2})^{2}}\, \mathrm{d}t \tag{$x = 1/(1+y)$} \\ &= \frac{1}{4} \int_{0}^{\infty} \frac{\mathrm{d}y}{\sqrt{y}(1+(1+y)^{2})} \tag{IbP} \\ &= \frac{1}{2} \int_{0}^{\inft..
블로그의 정체를 막기 위해, 이번에는 계산이 아니라 그냥 아주 간단한 내용을 올려봅니다. 방법론이 조금 제 마음에 들어서 올려봤는데, 너무 허접한 거 올렸다고 욕하진 마시고 그냥 감상해주세요 -ㅁ- 이 자리에서는 힐베르트 행렬 \begin{equation*} H_n = \begin{pmatrix} 1 & \frac{1}{2} & \cdots & \frac{1}{n} \\ \frac{1}{2} & \frac{1}{3} & \cdots & \frac{1}{n+1} \\ \vdots & \vdots & \ddots & \vdots \\ \frac{1}{n} & \frac{1}{n+1} & \cdots & \frac{1}{2n-1} \end{pmatrix} \end{equation*} 의 역행렬이 존재하고, 그..
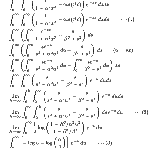 오늘의 계산 34 - Two Integrals
오늘의 계산 34 - Two Integrals
블로그의 정체를 막기 위해 오늘도 쉬운 계산 몇 가지를 적어보고자 합니다. [계산 1] α, β > 0 일 때 다음 계산이 성립합니다. 여기서 특별한 설명이 필요한 부분은 (1)에서 (4)에 해당하는 부분입니다. 우선 (1)은 제가 예전에 올린 글 중에서 적분의 교환가능성을 약간 확장한 것에 대한 글을 참조하시면 됩니다. 그리고 (2)와 (3)은 Lebesgue's dominated convergence theorem을 적용하면 되고, (4)는 감마함수의 미분으로부터 유도됩니다. [계산 2] 역시 쉬운 계산입니다. 오일러 적분의 일종이죠. 앗흥~
Problem. Evaluate the following integral. \begin{equation}\label{eqn:wts} \int_{0}^{1} \log (1-x) \log x \log (1+x) \; dx \end{equation} We divide the solution into several steps. 1. Reduction to Euler series. The key ingredient for the reduction is the following integral. \begin{equation*} \int_{0}^{\frac{\pi}{2}} \sin^{p} \theta \cos^{q} \theta \log \sin\theta \log \cos\theta \; d\theta. \end{..
(이 글은 스프링노트의 내용을 블로그로 옮기기 위한 테스트에 의해 작성되었습니다.) ...(전략)... 화사하던 2007년의 봄날, 화창한 햇빛이 쏟아지는 24동 2층의 한 강의실은 어떤 문제로 인해 봄철의 아지랑이보다 더욱 뜨거운 열기 속에 휩싸였다. 그 문제를 누가 처음 가져왔는지는 기억이 정확하지 않다. 다만 미적분학 과목 혹은 해석학 과목의 한 퀴즈 문제에서 다음과 같은 급수 가 수렴하는가를 묻는 문제가 나왔고, 당연히 대부분의 학생들은 시원스러운 답은커녕 수렴성 자체조차 가늠하지 못했다. 곧 이 문제는 tan-1의 오타로 인한 해프닝임이 밝혀졌다. 하지만 그 와중에 어느 누군가가 위의 급수 역시 절대수렴한다는 사실을 한 시간만에 증명해냈다는 이야기가 돌기 시작했고, 이는 이 문제를 향한 공략에 ..
 오늘의 계산 31 - An Integral (With Logarithmic Behavior)
오늘의 계산 31 - An Integral (With Logarithmic Behavior)
Problem. Evaluate the following integral. \begin{equation}\label{eqn:wts} \int_{0}^{1}\frac{\log \cos ( \frac{\pi x}{2} )}{x (x+1)} \, dx \end{equation} We first introduce a lemma which will play the key role to our calculation. Lemma. Define the function $F(s)$ by \begin{equation*} F(s) = \int_{0}^{1} \frac{\log | \cos (sx) | }{x} \, dx - \int_{1}^{2} \frac{\log | \sin (sx) | }{x} \, dx. \end{e..
요즘 리만제타함수 관련 내용을 공부하다가 제 취미랑 맞닥뜨리는 부분을 만나서 예전에 갖고 있던 관심이 다시 환기되 되살아났습니다. 그래서 구글링을 하면서 좀 관련 내용을 뒤지고 있는데, 여기 몇 가지 건진 파일들을 좀 올려놓으려고 합니다. [PDF] A CENTURY OF TAUBERIAN THEORY david borwein 1 D. Borwein, Tauberian theorems concerning Laplace transforms and. Dirichlet series, Arch. Math. ... G.H. Hardy and J.E. Littlewood, Tauberian theorems concerning power ... www.carma.newcastle.edu.au/~jb616/tauber..
- Total
- Today
- Yesterday
- 이항계수
- Integral
- 해석학
- 미쿠
- 적분
- 노트
- 푸리에 변환
- Euler integral
- 편미방
- 대수기하
- 계산
- 렌
- Zeta function
- 유머
- Beta function
- 보컬로이드
- Gamma Function
- Fourier Transform
- 제타함수
- 감마함수
- 수학
- Euler constant
- infinite summation
- Coxeter
- 오일러 상수
- 린
- 루카
- binomial coefficient
- 오일러 적분
- 무한급수
| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| 18 | 19 | 20 | 21 | 22 | 23 | 24 |
| 25 | 26 | 27 | 28 | 29 | 30 | 31 |


