수학 얘기/계산
오늘의 계산 13 - Integration Involved with Zeta Function
sos440
2008. 8. 13. 04:01
더운 방 안에 쳐박혀서 땀을 뻘뻘 흘리며 1시간 반 동안 계산한 끝에 얻은 결과입니다. 마치 프라모델광이 방에 쳐박혀 프라모델을 조립해 어엿한 1/100 MG 자×를 만들어내는 것과 비슷한 마음으로 풀었습니다. (이녀석, 위험하다... (˚;ε;˚;)a)
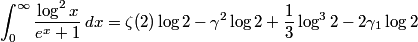
단, 여기서 γ1은 스틸체스 상수(Stieltjes constant) 입니다. 이런 상수가 있다는 건 알고 있었지만, 실제로 계산할 때에 바로 이 상수가 저 자리에 들어간다는 걸 깨닫지는 못했습니다. 그래서 이 상수의 값을 결정하기 위해 1시간동안 삽질을 했죠. 원래 제가 얻은 결과는
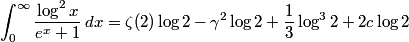
입니다. 단,
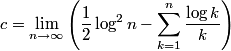
입니다. 처음 1시간동안은 c가 closed form으로 나타날 거라고 믿고 계산질을 했지만, 결과는 ㅇ<-<. 나머지 시간은 디리클레 에타함수와 리만 제타함수 사이의 관계성을 이용해서 c가 결국 -γ1임을 밝히는 데 사용했습니다.
이제 주어진 등식을 증명해봅시다. 일단 기본적인 실해석 지식과 감마, 제타함수의 기본적인 성질은 알고 있다고 가정하겠습니다. 또한 다음과 같은 사실들이 필요합니다.
Theorem 1 실수 s > 0 에 대해, 디리클레 에타함수(Dirichlet eta function)을
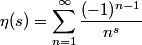
와 같이 정의하면, η는 무한 번 미분 가능하고, 그 n계 도함수는 위 무한급수의 각 항을 n번 미분하여 얻을 수 있다. 증명은 첨부파일 참조.
Theorem 2 f 가 [0, 1]위에서 정의된 C1함수이면, 다음 사실이 성립한다.

증명은 테일러 정리(Taylor's theorem)의 간단한 응용이므로, 생략한다.
Theorem 3 함수 I(s)를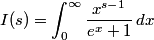 와 같이 놓으면,
와 같이 놓으면, 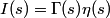 이 성립한다. 또한 I(s)의 n계 도함수는, 이 함수를 정의하는 적분식의 피적분함수의 s에 대한 n계 편미분을 적분한 결과와 같다. 증명은 각각 토넬리 정리(Tonelli's theorem)와 르벡 수렴정리(Lebesgue's dominated convergence theorem)의 응용이므로, 생략한다.
이 성립한다. 또한 I(s)의 n계 도함수는, 이 함수를 정의하는 적분식의 피적분함수의 s에 대한 n계 편미분을 적분한 결과와 같다. 증명은 각각 토넬리 정리(Tonelli's theorem)와 르벡 수렴정리(Lebesgue's dominated convergence theorem)의 응용이므로, 생략한다.
Theorem 4 감마함수와 관련하여, 폴리감마함수(polygamma function)를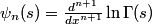 와 같이 정의하자. 그러면
와 같이 정의하자. 그러면 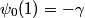 이고
이고 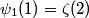 이다. 단,
이다. 단, 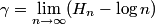 는 오일러-마스케로니 상수(Euler-Mascheroni constant)고
는 오일러-마스케로니 상수(Euler-Mascheroni constant)고 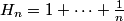 이다.
이다.
정리 1과 3을 이용하면, 우리가 구하려는 적분은 와 같음을 알 수 있으므로, 다음 식이 성립한다.
와 같음을 알 수 있으므로, 다음 식이 성립한다.
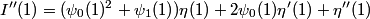
그러므로, η(1), η'(1), η''(1)의 값을 결정하기만 하면 원하는 적분의 값을 얻는다. 여기서 η(1)의 값을 구하는 것은 너무 쉬우므로 생략한다. 이때 만약
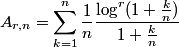
와 같이 두면, 다음 두 식이 성립한다.
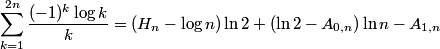
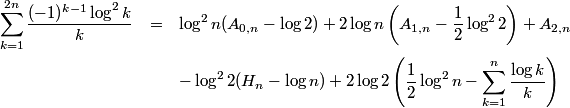
정리 2를 적용하면서 양 변에 n→∞ 의 극한을 취하면, 다음 두 식을 얻는다.
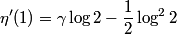

단, 여기서 c는 맨 앞에서 정의한 극한이며, 이 극한이 수렴함은 그래프를 통한 간단한 기하학적 고찰로도 충분히 확인할 수 있다. (물론, 순수히 해석적인 증명도 가능하다.) 그러므로 위 값들을 대입하여 계산하면, 최종적으로 우리가 원하는 적분의 값은
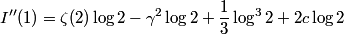
가 된다.
한편,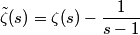 로 정의하면, 리만 제타함수와 디리클레 에타함수 사이의 간단한(정말로?) 관계에 의해 식
로 정의하면, 리만 제타함수와 디리클레 에타함수 사이의 간단한(정말로?) 관계에 의해 식 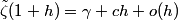 이 성립함을 알 수 있다. 그러므로 스틸체스 상수의 정의에 의해
이 성립함을 알 수 있다. 그러므로 스틸체스 상수의 정의에 의해 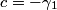 가 성립한다.
가 성립한다.
단, 여기서 γ1은 스틸체스 상수(Stieltjes constant) 입니다. 이런 상수가 있다는 건 알고 있었지만, 실제로 계산할 때에 바로 이 상수가 저 자리에 들어간다는 걸 깨닫지는 못했습니다. 그래서 이 상수의 값을 결정하기 위해 1시간동안 삽질을 했죠. 원래 제가 얻은 결과는
입니다. 단,
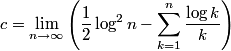
입니다. 처음 1시간동안은 c가 closed form으로 나타날 거라고 믿고 계산질을 했지만, 결과는 ㅇ<-<. 나머지 시간은 디리클레 에타함수와 리만 제타함수 사이의 관계성을 이용해서 c가 결국 -γ1임을 밝히는 데 사용했습니다.
이제 주어진 등식을 증명해봅시다. 일단 기본적인 실해석 지식과 감마, 제타함수의 기본적인 성질은 알고 있다고 가정하겠습니다. 또한 다음과 같은 사실들이 필요합니다.
Theorem 1 실수 s > 0 에 대해, 디리클레 에타함수(Dirichlet eta function)을
와 같이 정의하면, η는 무한 번 미분 가능하고, 그 n계 도함수는 위 무한급수의 각 항을 n번 미분하여 얻을 수 있다. 증명은 첨부파일 참조.
Theorem 2 f 가 [0, 1]위에서 정의된 C1함수이면, 다음 사실이 성립한다.

증명은 테일러 정리(Taylor's theorem)의 간단한 응용이므로, 생략한다.
Theorem 3 함수 I(s)를
Theorem 4 감마함수와 관련하여, 폴리감마함수(polygamma function)를
정리 1과 3을 이용하면, 우리가 구하려는 적분은
그러므로, η(1), η'(1), η''(1)의 값을 결정하기만 하면 원하는 적분의 값을 얻는다. 여기서 η(1)의 값을 구하는 것은 너무 쉬우므로 생략한다. 이때 만약
와 같이 두면, 다음 두 식이 성립한다.
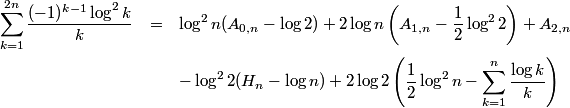
정리 2를 적용하면서 양 변에 n→∞ 의 극한을 취하면, 다음 두 식을 얻는다.
단, 여기서 c는 맨 앞에서 정의한 극한이며, 이 극한이 수렴함은 그래프를 통한 간단한 기하학적 고찰로도 충분히 확인할 수 있다. (물론, 순수히 해석적인 증명도 가능하다.) 그러므로 위 값들을 대입하여 계산하면, 최종적으로 우리가 원하는 적분의 값은
가 된다.
한편,
 invalid-file
invalid-file